Abstract
Hematopoietic stem cells (HSCs) replicate (self-renew) to create 2 daughter cells with capabilities equivalent to their parent, as well as differentiate, and thus can both maintain and restore blood cell production. Cell labeling with division-sensitive markers and competitive transplantation studies have been used to estimate the replication rate of murine HSCs in vivo. However, these methods are not feasible in humans and surrogate assays are required. In this report, we analyze the changing ratio with age of maternal/paternal X-chromosome phenotypes in blood cells from females and infer that human HSCs replicate on average once every 40 weeks (range, 25-50 weeks). We then confirm this estimate with 2 independent approaches, use the estimate to simulate human hematopoiesis, and show that the simulations accurately reproduce marrow transplantation data. Our simulations also provide evidence that the number of human HSCs increases from birth until adolescence and then plateaus, and that the ratio of contributing to quiescent HSCs in humans significantly differs from mouse. In addition, they suggest that human marrow failure, such as the marrow failure that occurs after umbilical cord blood transplantation and with aplastic anemia, results from insufficient numbers of early progenitor cells, and not the absence of HSCs.
Introduction
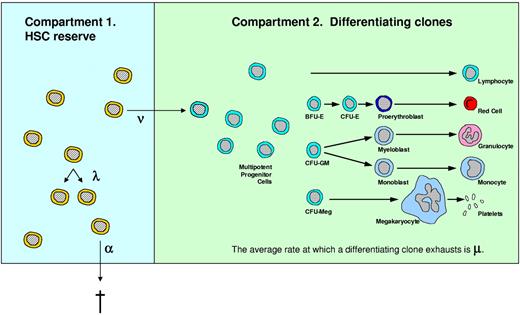
Hematopoiesis is a complex system. To ensure that blood cell production is robust (> 1011 red cells, granulocytes, and platelets produced each day) and durable (maintained throughout a lifetime), the parent cells, human hematopoietic stem cells (HSCs), are tightly regulated.1 Emerging data suggest that this is not accomplished by a uniform population of HSCs or by prescribed (robotic) decision-making. Rather, there are significant genetic and epigenetic differences among HSCs that drive fate decisions.2,3 The heterogeneity of HSCs makes it especially difficult to reconcile observations of individual cells with the behavior of hematopoiesis as a system. In addition, a cell's decisions can be modified by cytokines and by interactions with adjacent cells in its surrounding microenvironment,3,4 which adds further complexity. For these reasons, we have used a stochastic model to describe HSC decisions and to determine their consequences (Figure 1).
A stochastic model of hematopoiesis. The hematopoietic stem cell reserve (compartment 1) contains HSCs. Each HSC can replicate, differentiate, or die. Mean rates of HSC replication, differentiation, and HSC death (apoptosis) are denoted λ, ν, and α, respectively. Once an HSC commits to differentiation, it heads a clone that contributes mature blood cells for a finite period of time and then exhausts (mean rate μ). As HSCs act based on their unique intrinsic and microenvironmental signals, we assume that these fates are independent (the Markovian assumption). R0 and C0 are the numbers of HSCs and contributing (ie, short-term repopulating cell) clones at birth, respectively. The steady-state number of HSCs is termed K.
A stochastic model of hematopoiesis. The hematopoietic stem cell reserve (compartment 1) contains HSCs. Each HSC can replicate, differentiate, or die. Mean rates of HSC replication, differentiation, and HSC death (apoptosis) are denoted λ, ν, and α, respectively. Once an HSC commits to differentiation, it heads a clone that contributes mature blood cells for a finite period of time and then exhausts (mean rate μ). As HSCs act based on their unique intrinsic and microenvironmental signals, we assume that these fates are independent (the Markovian assumption). R0 and C0 are the numbers of HSCs and contributing (ie, short-term repopulating cell) clones at birth, respectively. The steady-state number of HSCs is termed K.
A stochastic analysis does not mean that the fate decisions of HSCs are random or chance occurrences. Rather this, and in particular Markovian analysis, is a tool for analyzing the dynamics of a system in which the decisions of the individual component(s) are so unique and/or complex that they cannot be completely observed or accurately and completely described, yet the joint (or average) behavior of the components acting collectively is predictable.5 These methods have been applied in diverse settings, such as describing how cars create traffic, how plants compete in ecosystems, and how cosmic rays hitting the atmosphere form cascades of elementary particles. They are amenable to describing how HSCs support hematopoiesis and specifically to defining the average rate, λ, at which HSCs replicate in vivo (Figure 1). By analyzing competitive transplantation studies,6-8 we have previously estimated λ for mouse and cat HSCs as once per 2.5 weeks and 8.3 weeks, respectively. Here, we apply similar stochastic methods to analyze the changing ratio of maternal/paternal X-chromosome phenotypes in blood cells of 1219 females 18 to 100 years of age (Montreal cohort) and 117 females 18 to 96 years of age (London cohort) to derive λ for human hematopoiesis. After confirming the reasonableness of this estimate by analyzing human marrow transplantation outcomes, we simulate human hematopoiesis to derive clinically relevant insights.
Methods
Use of X-chromosome inactivation ratios to track HSC replications
Because of X-chromosome inactivation during embryogenesis, somatic cells in females contain an active X-chromosome of either maternal origin or paternal origin. Because this process occurs randomly, the ratio of HSCs (and thus blood cells) with maternal versus paternal X-chromosome phenotypes should approximate 50:50. A 50:50 ratio is indeed seen at birth. However, with aging, this ratio progressively changes and the percentage of women with skewed hematopoiesis (defined as > 75% of blood cells expressing a single parental phenotype) increases.9-14 It is possible that some persons acquire a disorder, such as myelodysplasia, and their skewed hematopoiesis results from the clonal expansion of a single neoplastic stem cell.12,15 However, phenotypic skewing most probably results when HSCs that express the X-chromosome gene or genes of one parent have a subtle growth advantage over HSCs that express the X-chromosome gene(s) of the other parent and preferentially expand,10,12,13,16 a process termed hemizygous selection. Thus, by analyzing the drift of the X-chromosome phenotype of blood cells of human females with aging, one can gain information about relative replication rates of HSCs.
A similar drift of the X-chromosome phenotype of blood cells is seen with aging in female Safari cats, the F1 offspring of Geoffroy (South American origin) and domestic (Eurasian origin) cats.16 Geoffroy cats have evolved independently from domestic cats for 9 million years17 so that multiple polymorphisms genetically distinguish these animals, including polymorphisms on the X-chromosome that could provide cells with a subtle growth advantage. Using the description of hematopoiesis in Figure 1 and the value of λ derived from competitive transplantation studies (once per 8.3 weeks), we estimated that a 5% replication advantage for HSCs expressing X-chromosome alleles from the Geoffroy (vs domestic) parent would be sufficient to lead to the hemizygous selection of Geoffroy-type HSCs and explain these data.8
To analyze human hematopoiesis, we reasoned that X-chromosome polymorphisms in the outbred human population (only ∼ 150 000 years of genetic distance and chromosomal divergence18 ) would provide the same or fewer differences among HSC replication rates. With this single constraint, we analyzed data from Busque (Montreal cohort), which consists of 1219 observations in females 18 to 100 years of age (mean, 55.9 ± 22.0 years). Specifically, we simulated 1219 outcomes with an arbitrary λ and an arbitrary R0 (the number of HSCs at birth) using the description of hematopoiesis in Figure 1 and the other parameter values as described in “Defining K (the steady-state number of HSCs) and the parameter relationships.” For each simulation, we randomly drew replication rates for HSCs expressing the maternal X-chromosome and for HSCs expressing the paternal X-chromosome from a truncated normal distribution (mean λ), with variance chosen similar to the range observed in female Safari cats (Figure 2). In this way, we let the percentage difference between the domestic cat HSC replication rate λd and the Geoffroy cat HSC replication rate λG be approximate bounds on the percentage difference of the replication rates of human HSCs.
Choosing human HSC replication rates for simulations. For each simulated person, we selected the replication rates for HSCs expressing the maternal X-chromosome and for HSCs expressing the paternal X-chromosome at random from a distribution with common mean λ. The SD of this distribution was defined such that the ratio between values 2 SDs above and below this mean would be equal to the ratio between λd and λG in cats (the horizontal line). If the variability in humans is smaller than assumed here, there should be a narrower range of possible values for λ. We suspect that this more stringent limit (which would result in a narrower range of ∼ 1 per 40 weeks) is indeed probable.
Choosing human HSC replication rates for simulations. For each simulated person, we selected the replication rates for HSCs expressing the maternal X-chromosome and for HSCs expressing the paternal X-chromosome at random from a distribution with common mean λ. The SD of this distribution was defined such that the ratio between values 2 SDs above and below this mean would be equal to the ratio between λd and λG in cats (the horizontal line). If the variability in humans is smaller than assumed here, there should be a narrower range of possible values for λ. We suspect that this more stringent limit (which would result in a narrower range of ∼ 1 per 40 weeks) is indeed probable.
We then repeated this process 99 times and asked whether the simulated datasets (100 simulations of X-chromosome inactivation ratios in 1219 females) resembled the experimental data (Figure 3A-C) or not. If yes, then these λ and R0 values were included as possible values. If no, the combination was eliminated. The analysis was repeated for λ at increments of one per 5 weeks and for R0 at intervals of 100, so that full ranges of both parameters were assessed (a total of ∼ 100 million simulated persons, with each simulated dataset requiring 10-100 hours of computational time). We then analyzed data from Gale et al9 (London cohort), consisting of 117 observations in females 18 to 96 years of age (mean, 65.6 ± 23.5 years), with a comparable approach.
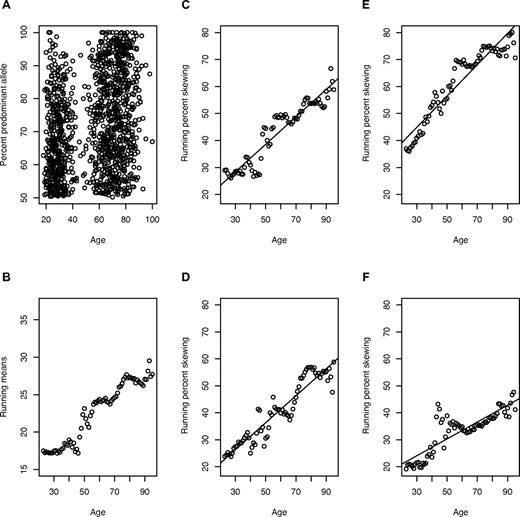
Estimating human replication rate through the analysis of changing maternal/paternal X-chromosome ratios with age in blood cells of females. (A) The percentage dominant allele for 1219 females 18 to 100 years of age. A value of 50% indicates no skewing, and 100% indicates complete skewing. (B-C) Means and percentages of persons with a skewed X-chromosome inactivation ratio (> 75% predominant allele), calculated using bin widths of 10 years. Means instead of the raw data were used to avoid overweighting more frequently occurring ages. (D-F) Percentage of persons with skewing in 3 sets of 100 simulations. For each simulation, we randomly drew replication rates for HSCs expressing the maternal X-chromosome and for HSCs expressing the paternal X-chromosome from a distribution (mean λ), with variance chosen similar to the range observed in Safari cats (Figure 2). The first simulations (D) use λ = 1 per 40 weeks and R0 (the number of HSCs at birth) = 300. Because R0 is uncertain, we considered this as a variable. The second set (E) uses λ = 1 per 20 weeks and R0 = 700, and the third (F) uses λ = 1 per 55 weeks and R0 = 300. To evaluate the appropriateness of R0 and λ, we compared the intercepts and slopes (representing value at birth and increase per year of age, respectively) for each of the regressions to the intercepts and slopes of the regressions for 100 sets of 1219 simulations (“Determining acceptable values of λ and R0”). Fitted regression lines are included. Parameter values in panel D yielded a similar y-intercept and slope to the observed data and were accepted; those in panels E and F did not and were rejected.
Estimating human replication rate through the analysis of changing maternal/paternal X-chromosome ratios with age in blood cells of females. (A) The percentage dominant allele for 1219 females 18 to 100 years of age. A value of 50% indicates no skewing, and 100% indicates complete skewing. (B-C) Means and percentages of persons with a skewed X-chromosome inactivation ratio (> 75% predominant allele), calculated using bin widths of 10 years. Means instead of the raw data were used to avoid overweighting more frequently occurring ages. (D-F) Percentage of persons with skewing in 3 sets of 100 simulations. For each simulation, we randomly drew replication rates for HSCs expressing the maternal X-chromosome and for HSCs expressing the paternal X-chromosome from a distribution (mean λ), with variance chosen similar to the range observed in Safari cats (Figure 2). The first simulations (D) use λ = 1 per 40 weeks and R0 (the number of HSCs at birth) = 300. Because R0 is uncertain, we considered this as a variable. The second set (E) uses λ = 1 per 20 weeks and R0 = 700, and the third (F) uses λ = 1 per 55 weeks and R0 = 300. To evaluate the appropriateness of R0 and λ, we compared the intercepts and slopes (representing value at birth and increase per year of age, respectively) for each of the regressions to the intercepts and slopes of the regressions for 100 sets of 1219 simulations (“Determining acceptable values of λ and R0”). Fitted regression lines are included. Parameter values in panel D yielded a similar y-intercept and slope to the observed data and were accepted; those in panels E and F did not and were rejected.
Additional information regarding subjects and specimens
All 1219 women in the Montreal cohort were of French-Canadian ancestry (with Quebec-born grandparents), answered a medical questionnaire, gave informed consent in accordance with the Declaration of Helsinki, and had normal blood counts. Participants were initially recruited for 2 different studies, one focusing on childbearing-age females11 and the other determining the effect of age on skewing in different hematopoietic and nonhematopoietic tissues (current study of L.B.), which explains the bimodal age distribution of subjects and the differences in sample processing. DNA was extracted from total blood for the younger cohort because the X-chromosome phenotype of T lymphocytes has been shown to correlate with that of granulocytes in younger persons.9 In the older cohort, granulocytes were separated from mononuclear cells by density gradient centrifugation (Ficoll-Paque, GE Healthcare). Data regarding the London cohort (females ages 18-50 and 75-96 years) are described by Gale et al.9 All studies were approved by the institutional review boards of all participating institutions.
Clonality assay methods and validation
X-chromosome inactivation (XCI) using the human androgen receptor assays (HUMARA) was performed as described previously.11 Briefly, the polymerase chain reaction amplification of the polymorphic CAG repeat at the HUMARA locus was realized in tandem on undigested (6-FAM–labeled primer) and on HpaII-digested (HEX-labeled primer) DNA. Amplification products were migrated on an ABI PRISM 3100 genetic analyzer (Applied Biosystems) to determine the area under the curve for each allele. XCI ratios are reported as percentage of predominant allele (Ppa): the percentage of the parental allele that is predominantly expressed ranges from 50% to 100%. For qualitative analysis purposes, to be consistent with prior reports,9-15 skewing was defined as a Ppa ≥ 75%, which corresponds to an XCI ratio ≥ 3:1.
To ensure accuracy, the reproducibility of results obtained with this XCI ratio determination method was evaluated by computing the “coefficient of repeatability,”19 which includes 95% of the differences between 2 repeated measurements. The coefficient of repeatability of XCI ratios was 0.033 (3.3%) for 1027 duplicate granulocyte samples. A sample known to be skewed was included in each polymerase chain reaction assay as a positive control. Analysis of 314 replicates of this control showed a mean Ppa score of 89% ± 7%.
Since others have suggested that acquired epigenetic changes can influence the results of X-inactivation–based assays that rely on differential methylation patterns, such as the HUMARA assay,20 Busque et al evaluated the blood samples from 100 elderly women and found that the results of this assay and 2 independent transcription-based clonality assays were concordant.14 Thus, if methylation changes do occur with aging, this should not significantly affect the results of X-chromosome inactivation determinations using HUMARA as performed in this study.
Defining K (the steady-state number of HSCs) and the parameter relationships
K is defined as 11 000 in all simulations (Figure 1). The derivation of K = 11 000 is discussed by Abkowitz et al,6 and it appears that K is similar for all mammals.21 Our previous experience indicates that the exact value of K, especially when K exceeds 500, minimally affects the simulated outcomes and rate calculations.
The values μ = 1 per 6.7 weeks, ν = .71λ, and α = .14λ are used in all calculations. Computer simulation results for mouse6 and Safari cat,7 and the more refined statistical methods feasible for the Safari cat analysis,22 estimate that μ (the rate at which short-term reconstituting cell–derived clones exhaust) approximates 1 per 6.7 weeks. Others, analyzing single-cell transplantations in mice23 and retroviral gene labeling in rhesus macaques,24 estimate μ as 1 per 4 to 12 weeks. That these mouse and macaque estimates overlap our mouse and cat estimates suggests that μ, like K, is conserved. Prior computer and statistical analyses6,7,22 also show that the model is relatively insensitive to the value of μ. In addition, simulations (not shown) demonstrate that μ = 1 per 10 weeks and μ = 1 per 12 weeks are acceptable values for human hematopoiesis.
Computer simulation results for Safari cats7 and mice6 indicate that the value of ν (the HSC differentiation rate) approximates 0.71 λ. Subsequent statistical refinements22,25 yield intervals that are substantially smaller using cat data and still consistent with this ratio. These computations are not possible using murine data because of the different experimental design, so that the ratio for murine hematopoiesis cannot be independently confirmed. However, others, with unrelated methods, have derived values of λ for mouse hematopoiesis26-29 that overlap (and thus support) our estimate of λ. Given these data, we adjust ν as λ is varied to maintain a ratio of 0.71. The stochastic model is indeed sensitive to this constraint because, for example, the hematopoietic stem cell reserve (compartment 1, Figure 1) will exhaust should ν exceed λ.
In contrast, with a fixed ratio of ν to λ, the model is not sensitive to changes in α (the HSC apoptosis rate; simulations not shown). The value of α ( = 0.14λ) was therefore computed by averaging the values from cat (0.17 λ)7 and mouse (0.11 λ)6 analyses. Additional mathematical details are in supplemental Methods (available on the Blood Web site; see the Supplemental Materials link at the top of the online article).
Defining the phenotype of HSCs at birth
The number of cells within R0 that express a maternal X-chromosome was modeled as a conditionally binomial random variable, recognizing that both X-chromosome inactivation and the selection of cells giving rise to hematopoiesis during fetal development are stochastic processes. Thus, the proportion of R0 HSCs expressing a maternal X-chromosome follows a binomial distribution with sample size R0 and random probability p, where p follows a binomial distribution with sample size 16 and success probability 0.5. Previous calculations16,30,31 (based on the variance in the G-6PD phenotype of feline and human blood cells at birth) estimate that 16 cells contribute to the origin of the definitive hematopoietic system. Because the replication rate of HSCs before birth is significantly faster than the replication rate of HSCs after birth,32,33 we let each initial cell have an equal chance of having an active paternal or maternal X-chromosome and then let the 16 cells proportionally increase in number until they reached R0.
Determining acceptable values of λ and R0
To capture the drift toward increased skewing with age, we calculated both running means and percentages of individuals with skewed X-chromosone inactivation ratio (>75% predominant allele; Figure 3B-C). We then compared intercepts and slopes for linear regressions fit to these values over time to intercepts and slopes of linear regressions fit to the simulated values. A total of 100 sets of 1219 simulations were run for values of λ in increments of 1 per 5 weeks and for R0 in increments of 100, producing 100 estimates of intercept and slope for each of the regressions which were used to derive empirical 95% confidence intervals (CIs). If the intercept and slope estimates from the linear regressions using the running means and percentage skewing calculated from the data all fell within the simulated 95% CIs for particular values of λ and R0, the values of λ and R0 used for those simulations were accepted. Particular values of λ were rejected only if there was no value of R0 that would reproduce 95% CIs for the 4 criteria (intercept and slope for each of running means and percentage skewing) covering the values obtained from the data. Were we to use only data for subjects younger than 75 years, the results would be similar but the empirical CIs would be larger, allowing for a somewhat wider range of possible values.
Calculating the time until R = K, the maximal capacity of the HSC reserve (compartment 1)
To calculate the time required to reach maximal capacity of the reserve, we set the theoretical (unbounded) mean R0 exp((λ − α − ν)t), where t is age, equal to 11 000 and solved for t. Because ν = 0.71 × λ and α = 0.14 × λ, we obtain t = (1 ÷ 0.15λ) × ln(11 000/R0). Although this mean calculation is inappropriate for the bounded model in general, it is (using a coupling argument34 ) appropriate for the growth stage.
Calculating the steady-state size of the contributing compartment (compartment 2)
The steady-state relationship between the first and second compartments is C/R ≈ v/(λ − α − v + μ).35
Results
Derivation and optimization of λ, the average replication rate of human HSCs
Despite broad criteria for comparableness (“Determining acceptable values of λ and R0”), λ of 1 per 20 weeks or faster and 1 per 55 weeks or slower failed to reproduce the Montreal data. This implies that human HSCs replicate once every 25 to 50 weeks in vivo (Figure 3). Acceptable values of R0, the number of HSCs at birth, for these λ, range from 200 to 11 000 (Table 1). To determine the “best” value of λ, we computed the replication rate permitting the greatest range of R0 and the rate producing the shortest CIs (Table 2). λ = 1 per 40 weeks with R0 = 300 HSCs appears optimal (Table 2).
The results were confirmed using the data from the London cohort. The acceptable values for the Montreal cohort (with one minor exception, λ = 1 per 35 weeks with R0 = 200) were also acceptable in analyses of this cohort. For the exceptional case, the simulated CI for the intercept of the running means failed to include the true value, although the endpoint was quite close; with R0 = 210 and higher, the CIs overlapped the data values.
Confirmation of λ using unrelated methodologies
Next, we compared this estimate to estimates of λ derived from the analysis of the mean telomere length of chromosomes in granulocytes with aging. Telomeres are end-chromosome tandem repeats of TTAGCG that progressively shorten with cell division. If the number of cell divisions between HSC and granulocyte is roughly constant, then the mean telomere length of chromosomes in granulocytes should reflect the mean number of self-renewal divisions that their HSC parents underwent before lineage commitment.36 Stochastic analyses using the single assumption that the ratio of HSC telomere shortening in vivo per cell division to T-cell telomere shortening in vitro per cell division is the same in cat and human, suggest λ = 1 per 45 weeks (range, 1 per 23-67 weeks),37 a value overlapping the results derived from studies of X-chromosome inactivation patterns.
For further confirmation, we estimated the replication rate for human HSCs, using the concept that the number of HSC replications in a mammal's lifetime is finite38 and conserved.36,37 A limited replication capacity and mandatory senescence would help preserve genetic integrity. An HSC in Safari cats replicates approximately once every 8.3 weeks,7,8 resulting in 94.0 replications during an average cat life span of 15 years.39 In C57BL/6J mice, HSCs replicate approximately once every 2.5 weeks,16,26,27 resulting in 58.9 HSC replications per average life span of 34.3 months.40 If the average human life span is 77 years,41 human HSC replication would occur between once per 43 weeks (simulating human hematopoiesis using replication limits from the cat data) and 67 weeks (from mouse data), or, averaging these, λ = 1 per 55 weeks. Thus, the estimates of the mean replication rate of human HSC with 3 independent methods, each making different assumptions, are remarkably similar.
Evidence that the hematopoietic system expands from birth to adolescence
We next computed the age at which a person's HSC compartment reaches its steady-state capacity using the theoretical mean size of the HSC reserve with no upper bound and estimating the time at which K = 11 000 is reached. Because telomeres shorten faster from birth to early infancy than later, it has been suggested that the HSC reserve must be complete by age 1 to 5 years.32 Using our best estimate for λ, once per 40 weeks, the reserve reaches 11 000 HSCs at 18.5 years, and for other plausible values of λ, capacity is reached at 9.3 to 20.8 years (Table 3). This argues that the number of HSCs in humans does not reach steady-state levels until adolescence, perhaps because the number of marrow niches expands with growth allowing increases in the numbers of HSCs.
Simulations with λ = 1 per 40 weeks accurately predict the outcomes of marrow transplantation
To explore the clinical implications of these results, we simulated blood cell production after HSC transplantation. We let λ = 1 per 40 weeks but varied R0 (the value of R at the start of the simulations, which here represents the number of donor HSCs) to predict the number of HSCs needed to sustain human hematopoiesis. When R0 = 10 HSCs, all of the simulations run out of active clones (cells in compartment 2 of Figure 1) within 30 weeks after transplantation, although HSCs (compartment 1 cells) still persist. When R0 = 20, 50% of the simulations run out of active clones (cells in compartment 2) within 30 weeks (75% run out of active clones within 100 weeks). However, when R0 = 100 or R0 = 200, no virtual transplantation recipient lacked active clones at any time point. The simulations thus suggest that a minimum of 100 HSCs should be transplanted (Table 4).
Given K = 11 000 and the estimate (from observing the distribution of 59Fe-transferrin among erythroid marrow cells43 ) that a 70-kg man has 1.5 × 1012 marrow cells, R0 = 100 is the number of HSCs present per 1.4 × 1010 marrow cells and would represent 1.9 × 108 marrow cells/kg if infused into a 70-kg recipient. This is concordant with the minimal safe marrow cell dose of 2 to 3 × 108 cells/kg recipient proposed for clinical transplantation.44
R0 = 20 (3.9 × 107 marrow cells/kg recipient) is a clinical situation where initial engraftment is doubtful and R0 = 50 (9.7 × 107 marrow cells/kg recipient) is a clinical situation where graft failure is common.44-46 The concordance of the simulated outcomes and clinical experience argues that λ = 1 per 40 weeks is a reasonable value.
As importantly, our data indicate that graft failure, such as that which occurs after umbilical cord blood transplantation or after transplantation with an inadequate number of marrow or peripheral blood stem cells, does not result from a lack or depletion of HSCs, but rather from an insufficient number of differentiating clones (Table 4; and data not shown). HSCs persist but do not differentiate fast enough or adequately to maintain blood cell production.
Evidence that human hematopoiesis differs from murine hematopoiesis
When simulation studies similar to that in Table 4 were done using the optimal parameter values for murine hematopoiesis (λ = 1 per 2.5 weeks, ν = 1 per 3.4 weeks, α = 1 per 20 weeks, and μ = 1 per 6.9 weeks6 ), different results were obtained. With R0 more than 30, all simulations successfully reconstituted and maintained hematopoiesis. With R0 = 20, only 3 (of 100) simulations failed to maintain blood cell production and with R0 = 10, 27 (of 100) failed. Failures occurred at 44 ± 17 weeks (range, 17-80 weeks) and interestingly always resulted from the absence of HSCs (compartment 1 cells in Figure 1), leading secondarily to the absence of contributing clones (compartment 2 cells). These data indicate that the kinetics of hematopoietic reconstitution after murine HSC transplantation differs from the kinetics of reconstitution after human HSC transplantation.
In addition, it appears that the steady-state ratio of the number of contributing clones to the number of quiescent HSC in humans differs from that in mouse. This ratio is estimated to be 1.46 for mice,6 0.677 for Safari cats,7,8 0.128 for baboons,47 and 0.116 for humans. Therefore, if K = 11 000, about 1275 HSC-derived clones contribute to human hematopoiesis and maintain steady-state blood cell production.
Discussion
Studying human hematopoiesis is challenging because the earliest components, HSCs, cannot be prospectively identified and observed. Here we use stochastic methods to define and characterize human hematopoiesis as a biologic system, and thus provide a method that complements traditional analyses. With this approach, we can analyze the outcomes of HSC decisions without mandating how or why these decisions occur.
Our data indicate that human HSCs replicate on average once per 40 weeks (range, 25-50 weeks) and, therefore, slower than nonhuman primate HSCs (∼ once per 25 weeks)47 and much slower than cat (∼ once per 8.3 weeks)7,8 or mouse (∼ once per 2.5 weeks)6 HSCs, when studied with comparable methods.
On the surface, this may seem surprising given the relative size and longevity of human versus mouse, but indeed, this ensures that the numbers of HSC replications per lifetime are reasonably similar,38,47 and thus minimizes the chances of untoward mutational events and of the accumulation of mutations in these critical parent cells. Interestingly, these replication kinetics allow for the expansion of the hematopoietic system after birth to occur at a pace such that the steady-state number of HSCs (K) is reached at adolescence, the time when the bony skeleton, and thus the marrow space and its microenvironment,4 reaches adult size.
To determine whether this estimate of the HSC replication rate were reasonable based on observations in humans, we modeled transplantation with differing numbers of HSCs and compared the simulated outcomes with clinical information.44-46 The data were remarkably concordant, supporting our stochastic methods and validating the estimates of K and λ. Simulated transplantations sometimes failed when 1 × 108 marrow cells/kg recipient were given to a virtual 70-kg recipient and were uniformly successful when the donor cell inoculum was more than 2 × 108 marrow cells/kg. Our data (Table 4) further suggest that the time to stable engraftment is a surrogate measure of HSC dose and thus may be a predictive and clinically useful measurement. This supports the clinical observations of Zubair et al42 that early neutrophil engraftment predicts long-term hematopoietic reconstitution by donor cells. These excellent correlations with clinical observations were seen without requiring that the division rate of HSCs increases immediately after transplantation when initial hematopoietic reconstitution occurs. The result holds, however (calculations not shown), if engrafting HSCs undergo an additional replication as a direct consequence of this stress, as has been predicted to occur in mouse.48
Importantly, the reserve of quiescent HSCs (compartment 1 in Figure 1) and numbers of contributing clones (compartment 2) were not replete for many years (even when 2-3 × 108 marrow cells/kg recipient are transplanted), consistent with the observation that patients remain unusually sensitive to chemotherapeutic agents long after HSC transplantation. Perhaps most interestingly, when graft failure occurred after the engraftment of small numbers of HSCs, this did not result from HSC depletion but rather from an insufficient number of contributing clones, so that hematopoiesis could not be adequately maintained while HSC regeneration was ongoing.
This latter observation has several clinically relevant corollaries. First, children (small size, fewer mature blood cells) tolerate transplantations of fewer HSCs better than adults because the few active clones can produce safe numbers of granulocytes, red cells, and platelets. Second, supplementing HSC transplantation with infusions of multipotent progenitor cells (such as present in ex vivo–expanded cord blood49 ) should be an efficacious method to ensure adequate hematopoiesis when HSC function is normal, HSC numbers are low, and the numbers of contributing clones are especially low. Third, our data raise the possibility that human marrow failure syndromes, such as aplastic anemia and myelodysplasia, could result from defective HSC commitment or the defective expansion of differentiating clones, and not only from the destruction or depletion of HSCs. Importantly, these events may be difficult to study in mice where simulations predict that graft failure is because of HSC depletion.
Taken together, stochastic analyses of HSC behavior will allow novel insights into hematopoiesis that complement and extend the results obtained with other experimental methods. In addition, the differences in HSC behavior between humans and mice justify the need for larger animal (eg, cat, dog, and primate) or human investigation.
The online version of this article contains a data supplement.
The publication costs of this article were defrayed in part by page charge payment. Therefore, and solely to indicate this fact, this article is hereby marked “advertisement” in accordance with 18 USC section 1734.
Acknowledgments
This work was supported by the National Institutes of Health (grants R01-HL46598 and R01-HL082933).
National Institutes of Health
Authorship
Contribution: S.N.C. and P.G. performed the stochastic analysis and modeling; J.L.A. directed the studies and provided the biologic analyses; S.N.C., P.G., and J.L.A. wrote the paper; and L.B. and R.E.G. obtained the patient data and edited the paper.
Conflict-of-interest disclosure: The authors declare no competing financial interests.
Correspondence: Janis L. Abkowitz, Division of Hematology, University of Washington, Box 357710, Seattle, WA 98195-7710; e-mail: janabk@u.washington.edu.